The first coordinate (X1 Y1 Z1) through a gap The second coordinate (X2 Y2 Z2) through a gap The third coordinate (X3 Y3 Z3) through a gap
Plane equation
Consider the problem of constructing a plane equation from points in space. This article is just the tip of the iceberg for calculating second-order surfaces in space. The same methodology is used as in the material. Calculation of a second-order curve on a plane
The equation of a plane in space has the form
It is easy to see that since there are three variables here, we uniquely determine all values of the plane by three points.
There are at least two ways to find the plane equation
1. We solve a vector product of the form
2. The solution of the matrix equation
And in both cases, the same answer is obtained, but the first option is more beautiful and visual like everything else in mathematics.
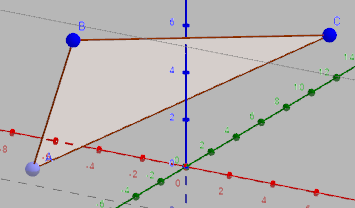
Check how it works
Let us be given three points with coordinates P 0 (1: -2: 0) P 1 (2: 0: -1) and P 2 (0: -1: 2)
Substituting the values in the equation we get.
Solving the equation, we get this result
In the case of a vector product, we need to solve the matrix
and we get
i%20+%20(%20-1%20)j%20+%20(%203%20)k%20+%20(%20-7%20)l)
That is, the same coefficients as in the first case
I would like to notice only one, three points that you will enter should not be on one straight line, since in this case, the equation of the plane cannot be calculated due to the ambiguity of its position in space.
Good luck!